Condensed Matter V

Quantum wells and heterojunctions

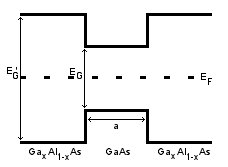
If we put two undoped semiconductors together, the chemical potential must be continuous across the junction- this creates a heterojunction. Two such junctions can be put together to form a quantum well.

In reality, this is a finite potential well, but we’ll treat it as an infinite one to make the maths easier.

Infinite potential well:

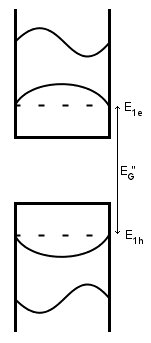Electrons have energy levels $E=\frac{\bar{h}^2n^2\pi^2}{2m_e*a^2}$ from the bottom of the conduction band.

Holes have energy levels $E=\frac{-\bar{h}^2n^2\pi^2}{2m_e*a^2}$ from the top of the valence band.

We now have an effective band gap E_G’’=E_G+E_1e+E_1h.

As we have confinement in one dimension, the system looks two-dimensional (when E₂-E₁>kT).

We get peaks in the absorption where there are transitions between two energy levels. As the well widens, the energy levels get closer and the absorption tends to the 3D absorption curve drawn earlier.

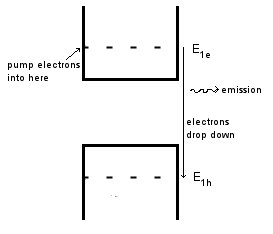
Quantum well laser: the laser transition is E₁ electrons to E₁ holes (these are the most populated levels, unsurprisingly). Quantum well lasers are used in fibre optic communications, CD players and laser pointers.

pn junction

Once again the chemical potential must be continuous across the junction. Say the potential across the gap $e\Delta V_0=E_G$

In the central region, electrons and holes annihilate to produce a depletion layer of low carrier density (ionised impurities are still present). There is a net E-field in this region.

pn junctions are massively useful.

Depletion layer: pn junction, area A.

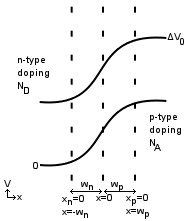
Potential across junction $e\Delta V_0=E_G$

Boundary conditions: E=0 at the edges of the depletion layer; E, V continuous at x=0 (x_n=w_n, x_p=-w_p)

Charge density on p-side $\rho_p=-eN_A$

Charge density on n-side $\rho_n=-eN_D$

$\nabla.E=\frac{\rho}{\epsilon_0\epsilon_r}$

Integrate and use boundary conditions to get

–w_n<x<0 $E=\frac{\rho_n x_n}{\epsilon_0\epsilon_r}=\frac{eN_Dx_n}{\epsilon_0\epsilon_r}$

0<x<w_p $E=\frac{\rho_p x_p}{\epsilon_0\epsilon_r}=\frac{-eN_Ax_n}{\epsilon_0\epsilon_r}$

At x=0, the E-field must be continuous, so N_Dw_n= N_Aw_p

Potential across depletion layer $V=\Delta V_0=-\int_{w_p}^{w_n}E dx$

–w_n<x<0 $V=\frac{eN_Dx_n^2}{2\epsilon_0 \epsilon_r}$

0<x<w_p $V=\frac{-eN_Ax_p^2}{2\epsilon_0 \epsilon_r}+\Delta V_0$

V must also be continuous at x=0

\frac{eN_Dx_n^2}{2\epsilon_0 \epsilon_r} =\frac{-eN_Ax_p^2}{2\epsilon_0 \epsilon_r}+\frac{E_G}{e}$

If N_D=N_A, w_n=w_p=δ, and the depletion layer has width 2δ

In this case

$\frac{-eN_A\delta^2}{\epsilon_0 \epsilon_r}+\frac{E_G}{e}$

$\delta^2=\frac{E_G\epsilon_0 \epsilon_r}{e^2N_D}$

δ~10^-6m

If δ is small, then tunnelling can occur.

Capacitance of a pn junction

Total stored charge Q=eN_Dw_nA

$\Delta V_0=\frac{eN_Dw_n^2}{2\epsilon_0 \epsilon_r}(\frac{N_A+N_D}{N_A}$ (we’re just using the equations above to express it in this form).

$\Delta V_0=\frac{Q^2}{2eN_DA^2\epsilon_0 \epsilon_r}(\frac{N_A+N_D}{N_A}$

$d(\Delta V_0)=\frac{QdQ}{eN_DA^2\epsilon_0 \epsilon_r}(\frac{N_A+N_D}{N_A})$

$C=\frac{dQ}{ d(\Delta V_0)}=\frac{eN_DA^2\epsilon_0 \epsilon_r}{Q}(\frac{N_A}{N_A+N_D})$

But recall that $N_D=\frac{w_p}{w_n}N_A$

So $C=\frac{A\epsilon_0\epsilon_r}{w_n}(\frac{1}{1+\frac{w_p}{w_n}}$

So $C=A\epsilon_0\epsilon_r(\frac{1}{w_n+w_p}$ which is the same form as we get for a parallel plate capacitor.

Usually, C≈100pF

Stored charge due to excess minority carriers on one side of a pn junction.

Continuity equation: rate of loss of carriers=recombination rate + outflow of carriers

$-\frac{\partial n}{\partial t}=\frac{n}{\tau}+\frac{\partial J}{\partial x}$

(for these purposes, J is a number current)

But $J=-D\frac{\partial n}{\partial x}$ , so

$\frac{\partial n}{\partial t}=-\frac{n}{\tau}+D\frac{\partial^2 n}{\partial x^2}$

In the steady state, $\frac{\partial n}{\partial t}=0$

$\frac{\partial^2 n}{\partial x^2}=\frac{n}{D\tau}$

$n=n_0e^{\frac{-x}{\sqrt{D\tau}}}$

Define a diffusion length $l=\sqrt{D\tau}$

$n=n_0e^{\frac{-x}{l}}$

At, x=0, $J=\frac{Dn_0}{l}$ – total current at x=0

Total carrier number $N=\int_0^{\infty}n_0e^{\frac{-x}{l}}dx=n_0l$

$J=\frac{DN}{l^2}=\frac{N}{\tau}$

Total stored charge $Q=eN=eJ\tau=I\tau=\frac{Il^2}{D}$

pn junction with an applied bias (Ebers-Moll equation)

No bias

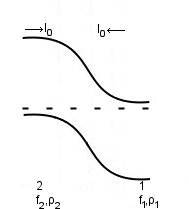
Fermi Golden Rule

right -> left $R_{\uparrow}=\frac{2\pi}{\bar{h}}|M|^2\rho_1f_1\rho_2(1-f_2)$

left -> right $R_{\downarrow}=\frac{2\pi}{\bar{h}}|M|^2\rho_2f_2\rho_2(1-f_1)$

If f₁=f₂, these are identical and there is no net current.

Apply a bias (voltage) across the junction.

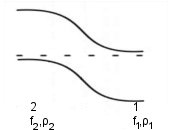
Raise energy levels on RHS

Now $f_1=f_2e^{\frac{eV}{kT}}$ forward bias

$R_{\uparrow}\propto\rho_1\rho_2f_1(1-f_1e^{\frac{eV}{kT}})$

$R_{\downarrow}\propto\rho_1\rho_2(1-f_1)f_1e^{\frac{eV}{kT}}$

These rates are proportional to the current I.

Total

$I \propto [f_1(1-f_1 e^{\frac{eV}{kT}})-(1-f_1)f_1 e^{\frac{eV}{kT}}]$

$I \propto f_1-f_1^2 e^{\frac{eV}{kT}}-f_1 e^{\frac{eV}{kT}}+f_1^2 e^{\frac{eV}{kT}}]$

$I=I_0[1- e^{\frac{eV}{kT}}]$ Ebers-Moll equation

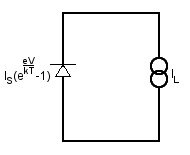
Solar cell: forward biased pn junction.

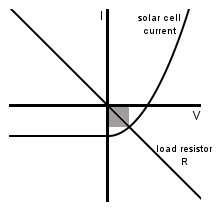
The shaded box represents the power output of the solar cell.

The solar cell has a total current $I=I_S(e^{\frac{eV}{kT}}-1)-I_L$

Looks like a diode and a constant current source.

Einstein relation

Electrons $\frac{D_e}{\mu_e}=\frac{kT}{e}$

Holes $\frac{D_h}{\mu_h}=\frac{kT}{e}$

The D‘s are just diffusion constants.

Potential across a pn junction (revisited)

Start by thinking about total currents (be careful of sign conventions in this section)

Electrons $J_e=nq\mu E-qD\frac{dn}{dx}$

$J_E=nq\mu\frac{dV}{dx}-qD\frac{dn}{dx}$

and similarly for holes.

In equilibrium, J=0

$\frac{dV}{dx}=\frac{-D}{\mu n}\frac{dn}{dx}$

$int_0^{v_0}dV=\frac{-kT}{e}\int_{n_D}^{\frac{n_i^2}{N_A}}\frac{dn}{n}$

(we get the limits by remembering that on the n-side, $n=N_D$ and that from the Law of Mass Action, on the p-side, $n=\frac{n_i^2}{N_A}$ )

Integrate to get $\Delta V_0=\frac{kT}{e}\ln(\frac{N_DN_A}{n_i^2})$

pn junction breakdown at large reverse bias can occur in two ways:

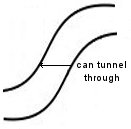
Tunnelling: a valence electron in the p-region makes a transition to the conduction band of the n-region. Tunnelling requires N_D, N_A ~5×10²³ m^-1 -> E > 10⁸ Vm^-1
Avalanche: a thermally generated electron gains enough kinetic energy in the E-field to cause impact ionisation in which an electron-hole pair is generated. Multiplication factors of ~100 can be achieved with several hundred volts of reverse bias.

Photodiodes are used to measure optical signals. There are two modes of operation.

Photovoltaic mode: photons with hν>E_G generate electron-hole pairs in a pn junction. Electrons are swept to the positively charged n region and holes to the negatively charged p region, reducing the voltage below its equilibrium value.

Current generated by a beam of light, power P, photon energy hν

$I_p=\frac{q\eta P}{h\nu}$ where η is the fraction of photons that generate an electron-hole pair.

New drift current=I₀+I_P

To maintain equilibrium, diffusion current must also increase.

Change in junction voltage is ΔV_J

Diffusion current is now $I_D=I_0e^{\frac{q\Delta V_J}{kT}}$

$I_0+I_P= I_0e^{\frac{q\Delta V_J}{kT}}$

$\Delta V_J=\frac{kT}{q}\ln(\frac{I_P}{I_0}+1)$

Photoconductive mode: a reverse bias is applied to the junction, reducing the drift and diffusion currents and increasing the width of the depletion layer. If photons are now absorbed in the depletion layer, a ‘generation’ current is produced which is proportional to the absorbed power.

Sensitivity $R_0=\frac{I_P}{P}=\frac{q\eta}{h\nu}$

η~0.8, R₀~0.55 AW^-1

We can improve the sensitivity by incorporating a relatively thick intrinsic layer between the two doped regions so that more of the incident light is absorbed. This is a p-i-n diode.

Speed of a photodiode detector depends on

Drift time of carriers across the depletion layer.
Diffusion through p and n regions (diffusion is slower than drift, so those layers must be much thinner than the depletion layer).
RC time constant of the circuit.

Solar cell revisited: current through a solar cell $I=I_S(e^{\frac{qV}{kT}}-1)-I_L$

Open circuit voltage (I=0)

$I_S(e^{\frac{qV_{OC}}{kT}}-1)=I_L$

$e^{\frac{qV_{OC}}{kT}}=\frac{I_L}{I_S}+1$

$V_{OC}=\frac{kT}{q}\ln(\frac{I_L}{I_S}+1)$ looks familiar, doesn’t it?

Physics Made Easy